The Ball Corporation's beverage can manufacturing plant in Fort
Atkinson, Wisconsin, uses a metal supplier that provides metal with a
known thickness standard deviation σ = .000619 mm. Assume a
random sample of 46 sheets of metal resulted in an x¯ = .1903
mm.
Calculate the 99 percent confidence interval for the
true mean metal thickness. (Round your answers to 4 decimal places.)
.1903±2.576(.000619/√46)=.1903±.0002351 or (.1901,.1905)
Last year, 10 percent of all teenagers purchased a new iPhone. This year, a sample of 260 randomly chosen teenagers showed that 39 had purchased a new iPhone. The test statistic to find out whether the percentage has risen would be
p = 39/260 = .15,
π 0 = .10,
z calc = (.15 − .10)/[(.10)(1 −
.10)/260]1/2 = 2.68742
A study showed that 14 of 180 publicly traded business services companies failed a test for compliance with Sarbanes-Oxley requirements for financial records and fraud protection. Assuming that these are a random sample of all publicly traded companies, construct a 95% confidence interval for the overall noncompliance proportion. (Round your answers to 4 decimal places.)
p=14/180=.0778.
p±z√(p(1−p)/n)
.0778±1.96√(.0778(1−.0778)/180)
=.0778±.0391 or (.0387, .1169)
A random sample of 25 items is drawn from a population whose standard
deviation is known to be σ = 40. The sample mean is x−x− =
270.
(a) Construct an interval
estimate for μ with 95 percent confidence. (Round
your answers to 2 decimal places.)
(b) Construct an interval estimate for μ with 95 percent confidence assuming that n = 50. (Round your answers to 2 decimal places.)
(c) Construct an interval estimate for μ with 95 percent confidence assuming that n = 100. (Round your answers to 2 decimal places.)
(d) Describe how the confidence interval changes as n increases.
a) 270±1.96(40/√25)
=270±15.68 or (254.32, 285.68)
b) 270±1.96(40/√50)
=270±11.09 or (258.91, 281.09)
c) 270±1.96(40/√100)
=270±7.84 or (262.16,277.84)
d) Width decreases as n increases
A manufacturer claims that its compact fluorescent bulbs contain an
average of 1.2 mg of mercury.
State the hypotheses for a
two-tailed test, using the manufacturer’s claim about the mean as the
null hypothesis.
H 0: μ = 1.2 mg vs. H 1: μ ≠ 1.2 mg
A survey of 4,581 U.S. households that owned a mobile phone found that 58 percent are satisfied with the coverage of their cellular phone provider. Assuming that this was a random sample, construct a 90% confidence interval for the true proportion of satisfied U.S. mobile phone owners. (Round your answers to 3 decimal places.)
p±z√(p(1-p)/n)
.58±1.645√(.58(.42)/4581)
= .58±.0120 = (.568, .592)
The process that produces Sonora Bars (a type of candy) is intended to produce bars with a mean weight of 56 gm. The process standard deviation is known to be 0.77 gm. A random sample of 49 candy bars yields a mean weight of 55.82 gm. Find the p-value for a test to see whether the candy bars are smaller than they are supposed to be.
a) Between .05 and .10
b) Less than .01
c) Between .01
and .025
d) Between .025 and .05
a) Between .05 and .10
z calc = (55.82 − 56)/[(0.77)/491/2] = −1.63636
and z .05 = −1.645,
or find the exact p-value as =NORM.S.DIST(-1.63636,1) = .0509.
Construct a confidence interval for μ assuming that each sample is from a normal population.
(a) x¯x¯ = 30, σ = 5, n = 13, 90 percentage confidence. (Round your answers to 2 decimal places.)
(b) x¯x¯ = 124, σ = 10, n = 27, 99 percentage confidence. (Round your answers to 2 decimal places.)
(c) x¯x¯ = 12.1, σ = 1.1, n = 50, 95 percentage confidence. (Round your answers to 3 decimal places.)
a) 30 ± 1.645(5/√13) = 30 ± 2.28
or (27.72, 32.28)
b) 124 ± 2.576(10/√27) = 124 ± 4.96
or (119.04, 128.96)
c) 12.1 ± 1.96(1.1/√50) = 12.1 ± .305
or (11.795, 12.405)
In a recent survey, 10 percent of the participants rated Pepsi as
being "concerned with my health." PepsiCo's response
included a new "Smart Spot" symbol on its products that meet
certain nutrition criteria, to help consumers who seek more healthful
eating options.
Suppose a follow-up survey shows that 28
of 200 persons now rate Pepsi as being "concerned with my
health."
Calculate the z statistic.
(Round your answer to 3 decimal places.)
At α = .05, would a follow-up survey showing that 28 of 200 persons now rate Pepsi as being “concerned with my health” provide sufficient evidence that the percentage has increased?
a) H 0: π ≤ .10 vs. H 1: π > .10.
z= (.1400-.10)/√(.10(.90)/200)
z = 1.886
b) Yes
p-value = 1 – NORM.S.DIST(1.886,1) = 1 – .9704 = .0296 and .0296 < .05, so reject H 0 and conclude that the proportion who believe Pepsi is concerned with consumers’ health has increased.
The average age of a part-time seasonal employee at a Vail Resorts
ski mountain has historically been 37 years.
State the
hypotheses one would use to test if this average has decreased since
the last season.
a) H 0: μ ≥ 37 yrs vs. H 1: μ < 37 yrs
b) H 0: μ ≤ 37 yrs vs. H 1: μ > 37 yrs
c) H 0: μ = 37 yrs vs. H 1: μ ≠ 37 yrs
a) H0: μ ≥ 37 yrs vs. H1: μ < 37 yrs
In a two-tailed test, a statistician got a z test statistic of 1.82. What is the p-value?
a) .0708
b) .0688
c) .0301
d) .0874
b) .0688
From Appendix C we get 2 × P(Z > 1.82) = 2 × .0344 = .0688. The Excel version of this calculation is =2*(1-NORM.S.DIST(1.82,1)) = 0.0688.
In the nation of Gondor, the EPA requires that half the new cars sold will meet a certain particulate emission standard a year later. A sample of 64 one-year-old cars revealed that only 24 met the particulate emission standard. The test statistic to see whether the proportion is below the requirement is
a) -1.645
b) -2.000
c) -1.960
d) -2.066
b) -2.000
p = 24/64 = .375, π 0 = .50, z calc = (.375 − .50)/[(.50)(1 − .50)/64]1/2 = −2.000.
(a) A poll of 2,237 likely voters was conducted on the president’s performance. Approximately what margin of error would the approval rating estimate have if the confidence level is 95%? (Round your answer to 4 decimal places.)
(b) The poll showed that 46 percent approved the president’s performance. Construct a 90 percent confidence interval for the true proportion. (Round your answers to 4 decimal places.)
(c) Would you agree that the percentage of all voters opposed is likely to be 50 percent?
a) .0210
Margin of error = 1.960 √(.50(1-.5)/2237)
= .0207
b) (.4425, .4775)
p±z√(p(1−p)/n)
=0.46±1.645√(0.46(1 −0.46)/2,237)
=0.46±0.0173 or (0.4427, 0.4773)
c) No, the confidence interval does not contain .50.
Last year, 10 percent of all teenagers purchased a new iPhone. This year, a sample of 260 randomly chosen teenagers showed that 39 had purchased a new iPhone. To test whether the percentage has risen, the p-value is approximately
a) .0501
b) .0314
c) .0492
d) .0036
d) .0036
p = 39/260 = .15, π 0 = .10, z calc = (.15 − .10)/[(.10)(1 − .10)/260]1/2 = 2.68742. From Appendix C we get P(Z > 2.69) = .0036, or from Excel =1-NORM.S.DIST(2.68742,1) = .0036.
The process that produces Sonora Bars (a type of candy) is intended to produce bars with a mean weight of 56 gm. The process standard deviation is known to be 0.77 gm. A random sample of 49 candy bars yields a mean weight of 55.82 gm. Find the test statistic to see whether the candy bars are smaller than they are supposed to be.
a) -1.677
b) -1.645
c) -1.636
c) -1.636
z calc = (55.82 − 56)/[(0.77)/491/2] = −1.63636.
In a sample of 679 new websites registered on the Internet, 42 were
anonymous (i.e., they shielded their name and contact
information).
(a) Construct a 95
percent confidence interval for the proportion of all new websites
that were anonymous. (Round your answers to 4 decimal places.)
(b) May normality of p be assumed?
a) (.0437, .0800)
p = 42/679 = .06186
p±z√(p(1-p)/n)
.06186 ± 1.960 √.06186(1-.06186)/679)
= .06186 ± .0181 or (.0437, .0800)
b) Yes
A random sample of 150 items is drawn from a population whose standard deviation is known to be σ = 40. The sample mean is x¯ = 790.
(a) Construct an interval estimate for μ with 99 percent confidence. (Round your answers to 1 decimal place.)
(b) Construct an interval estimate for μ with 99 percent confidence, assuming that σ = 80. (Round your answers to 1 decimal place.)
(c) Construct an interval estimate for μ with 99 percent confidence, assuming that σ = 160. (Round your answers to 1 decimal place.)
(d) Describe how the confidence interval changes as σ increases.
a) (781.6, 798.4)
790 ± 2.58 (40/√150)
b) (773.2, 806.8)
790 ± 2.58 (80/√150)
c) (756.3, 823.7)
790 ± 2.58 (160/√150)
d) The confidence interval gets wider as σ increases.
A firm decides to test its employees for illegal drugs.
(a) State the null and alternative hypotheses.
(b) Define Type I and II errors.
(c) In this case, which type of error has the more severe consequences?
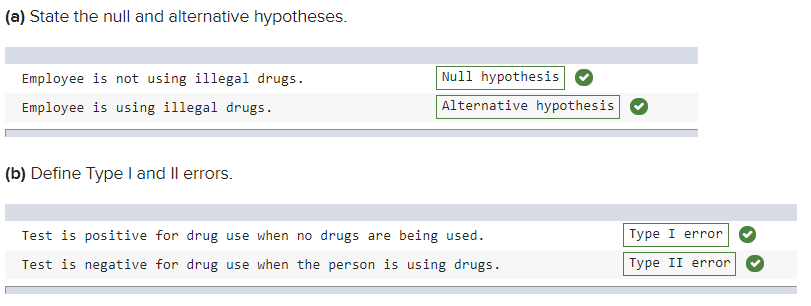
a&b in image attachment
c) Type II error
A) Choose the correct set of hypotheses for a two-tailed test for a zero slope.
a) H 0: β 1 ≥ 0 vs. H 1: β 1 < 0
b) H 0: β 1 ≤ 0 vs. H 1: β 1 > 0
c) H 0: β 1 = 0 vs. H 1: β 1 ≠ 0
B-1) What is the p-value? (Round your answer to 4 decimal places.)
B-2) State the 95% confidence interval for the slope.
C) Choose the correct statement.
-The slope is not significantly different from zero.
-The slope is significantly different from zero.
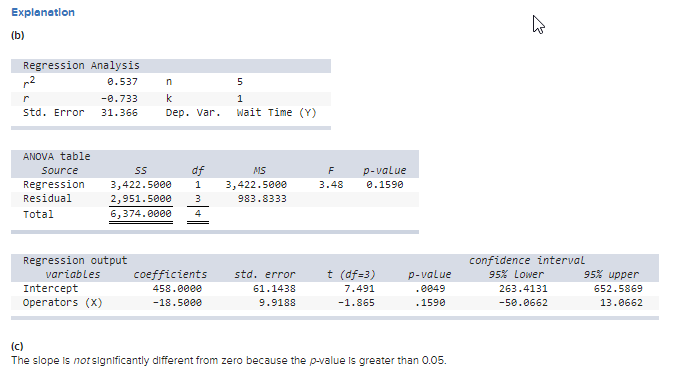
A) c) H 0: β 1 = 0 vs. H 1: β 1 ≠ 0
B-1) .1590
B-2) The confidence interval is from -50.0662 to 13.0662
C) The slope is not significantly different from zero.
The fitted regression is Sales = 902-32.8(Price)
A-1) If Price = 1, what is the prediction for Sales? (Round your answer to 1 decimal place.)
A-2) Choose the correct statement.
- An increase in price increases sales.
- A decrease in price decreases sales.
- An increase in price decreases sales.
B) If Price = 22, what is the prediction for Sales? (Round your answer to the nearest whole number.)
C) Choose the right option
- The intercept is not meaningful as a zero price is both unrealistic and unobserved. Correct
- The intercept is meaningful as sales will be maximized when price is zero.
A-1) Sales = 902-1(32.8) = 869.2
A-2) An increase in price decreases sales.
B) Sales = 902 - 22(32.8) = 180
C) The intercept is not meaningful as a zero price is both unrealistic and unobserved.
Use Excel to calculate the sample correlation coefficient for each data set. (Round your answers to 4 decimal places.)
Put each data set into excel and use CORREL(X Data, Y Data)
A) Choose the right option
- An increase in orders leads to an average decrease in shipping cost.
- An increase in orders leads to an average increase in shipping cost.
B) Choose the correct statement.
- The intercept is not meaningful in this case. Correct
- The intercept is meaningful in this case.
C) Calculate R 2. (Round your answer to 4 decimal places.)
D) Interpret the R 2. (Round your answer to 4 decimal places.)
A) An increase in orders leads to an average increase in shipping cost.
B) The intercept is not meaningful in this case.
C) .6717
D) 67.17%
The regression equation NetIncome = 2,185 + .0392 Revenue was estimated from a sample of 100 leading world companies (variables are in millions of dollars).
(a-1) Calculate the residual for the x, y pair ($44,681, $3,720). (A negative value should be indicated by a minus sign. Round your answer to 4 decimal places.)
- The regression equation overestimated the net income.
- The regression equation underestimated the net income.
(b-1) Calculate the residual for the x, y pair ($63,662, $4,513). (A negative value should be indicated by a minus sign. Round your answer to 4 decimal places.)
(b-2) Did the regression equation underestimate or overestimate the net income?
- The regression equation overestimated the net income.
- The regression equation underestimated the net income.
a-1)y^= $2,185 + .0392($44,681) = $3,936.4952
ei = 3,720
– 3,936.4952 = –216.4952
a-2) The regression equation overestimated the net income.
b-1) y^ = $2,185 + .0392($63,662) = $4,680.5504
ei = 4,513 – 4,680.5504 = –167.5504
b-2) The regression equation overestimated the net income.
Choose the dependent variable (the response variable to be
"explained") and the independent variable (the
predictor or explanatory variable).
Dependent Variable
- ELOS
- ALOS
Independent Variable
- ALOS
- ELOS
Obtain the regression equation. (Round your answers to 3 decimal places.)
Calculate R 2
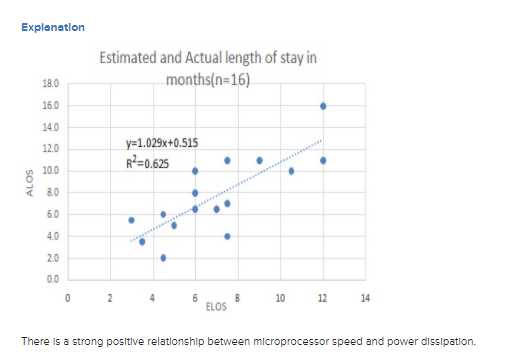
Dependent Variable = ALOS
Independent Variable = ELOS
Regression Equation = Y = 1.029X + .515
R 2 = .625
Researchers found a correlation coefficient of r = .50 on
personality measures for identical twins. A reporter interpreted this
to mean that "the environment orchestrated one-half of their
personality differences."
(a) r is a measure of the strength and direction of
the linear relationship.
- No
- Yes
(b) r is a measure of the amount of variation.
- Yes
- No
(c) r = 0.5 implies the environment orchestrated one-half of their personality differences.
- True
- False
a) Yes
b) No
c) False
Explanation
No, r measures the strength and direction of the linear relationship, but not the amount of variation explained by the explanatory variable.
Which is not revealed on a scatter plot?
- Nonlinear relationships between X and Y
- Missing data values due to nonresponses
- Pairs of observed (x i, yi) data values
- Unusual data values (outliers)
Missing Data values due to nonresponses.
Explanation
Excel simply omits missing data for a scatter plot.
Simple regression was employed to establish the effects of childhood
exposure to lead. The effective sample size was about 122 subjects.
The independent variable was the level of dentin lead (parts per
million). Below are regressions using various dependent
variables.
(a) Calculate the t statistic for each slope. From
the p-values, which slopes differ from zero at α = .01? (Round
your answers to 2 decimal places. Negative values should be
indicated by a minus sign.)
(b) It would be inappropriate to assume cause and
effect without a better understanding of how the study was conducted.
- No
- Yes
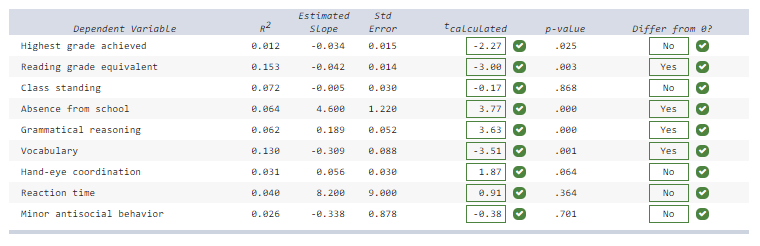
(a) answers in image
t=
estimated slope
standard error
(b) Yes